Return to Main Index page          Go to Lecture 16         Go to Lecture 17 

Go to movie of discrete logistic 2-cycle (r = 2.2) case       Go to movie of discrete logistic converge-to-K (r = 1.5) case

Intraspecific competition -- discrete logistic equation
very high (chaotic) r case

                  Eqn 15.1

Embedded QuickTime Movie (from Mathematica notebook ChaosMovie.nb)

Click on the image below to start the "movie". A control bar will appear below the graph; the various buttons will allow you to stop and start it.

Fig. 1. One-dimensional "map" of the discrete logistic (Eqn 15.1, above) with r = 3 and K = 1,000. Note that the population never settles on any value or set of values. We start with an N(t) value on the X-axis, move up to the "map" (red line) to get an N(t+x) value and then take the corresponding Y-value by going over to the 1:1 black line, then again move to the red line. Tracing the map is therefore a process of going back and forth from the red map line to the black N(t+x) = N(t) 1:1 line (like a box step dance -- "up, right, down, left"). Following the map this way gives us the successive population sizes over time. Note that the trajectory moves away from the unstable equilibrium point (at K = 1,000) and toward a chaotic pattern of highly variable population sizes.  Here, the "movie" runs for 99 time steps.  We could go for much longer without ever repeating our steps. 

The high r value here is what drives the cycle.

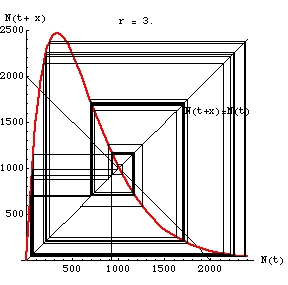
Fig. 2. Static view of the trajectory for 99 time steps in the chaotic range from a starting N₀ of 950. 
Note that values near unstable equilibrium point K (1,000) will spiral outward.  The places where the values spend more time (and develop thicker black lines) are called "strange attractors" in chaos theory.