Return to Main Index page          Go to Lecture 16         Go to Lecture 17  

      Go to movie of discrete logistic chaos (r = 3) case           Go to movie of discrete logistic converge-to-K (r = 1.5) case

Intraspecific competiton -- discrete logistic equation
      high r 2-point cycle case

                  Eqn 15.1

Embedded QuickTime Movie (from Mathematica notebook DiscLogAnimTraj03.nb)

Click on the image below to start the "movie". A control bar will appear below the graph; the various buttons will allow you to stop and start it.

Fig. 1. One-dimensional "map" of the discrete logistic (Eqn 15.1, above) with r = 2.2 and K = 1,000. Note that the population converges on a two-point cycle. We start with an N(t) value on the X-axis, move up to the "map" (red line) to get an N(t+x) value and then take the corresponding Y-value by going over to the 1:1 black line, then again move to the red line. Tracing the map is therefore a process of going back and forth from the red map line to the black N(t+x) = N(t) 1:1 line (like a box step dance -- "up, right, down, left"). Following the map this way gives us the successive population sizes over time. Note that the trajectory moves away from the unstable equilibrium point (at K = 1,000) and toward an oscillation between two other stable equilibrium points -- one at 497 and the other at 1,503. Once it gets there it will oscillate endlessly between these two values.

The high r value here is what drives the cycle.

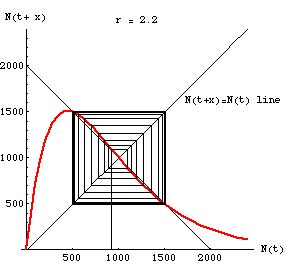
Fig. 2. Static view of the trajectory toward the 2-point stable equilibrium from a starting N₀ of 950. 
Note the rectangular outward spiral from the starting value (near the unstable equilibrium point K) to the stable oscillatory points, 497 and 1503.  The upper left corner is at {497, 1503} while the lower right is {1503, 497}. These are the two places the red map curve crosses the line of slope -1 (it also crosses at K).